• Real Analysis
• 1. Sets and Relations
• 2. Infinity and Induction
• 3. Sequences of Numbers
• 4. Series of Numbers
• 5. Topology
• 6. Limits, Continuity, and Differentiation
• 7. The Integral
• 7.1. Riemann Integral
• 7.2. Integration Techniques
• 7.3. Measures
• 7.4. Lebesgue Integral
• 7.5. Riemann versus Lebesgue
• 8. Sequences of Functions
• 9. Historical Tidbits
• Java Tools

Example 7.3.3(b): Outer Measure of Intervals

Why these ads ...

Since outer measure should be related to 'length' we expect that the outer measure of [a, b] is b - a. Indeed, that is the case.

Take the interval (a - , b + ). That interval covers [a, b] so that

m^*([a, b]) b - a + 2

Since was arbitrary we have

(*)      m^*([a, b]) b - a

To show the other inequality, we must show that for any collection I_n of intervals whose union covers [a, b] we have

l(I_n) b - a

Take such a collection and assume, for the moment, that it is finite. Order that collection as follows:

• The first interval is the one that contains a. Call it (a₁, b₁).
• If b₁ < b, then pick as the second interval that which contains b₁. Call it (a₂, b₂).
• If b₂ < b, then pick as the third interval that which contains b₂. Call it (a₃, b₃).

Continue in this fashion until either one of the b_j > b or until all intervals are numbered. Since there are only finitely many open intervals, the process must terminate in finitely many steps, say in k steps, and since the intervals cover [a, b] we must have that b_k > b.

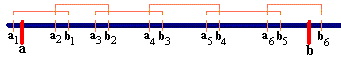
Subintervals with k = 6

Then

l(I_n) (b₁ - a₁) + (b₂ - a₂) + ... + (b_k - a_k) =
      = -a₁ + (b₁ - a₂) + (b₂ - a₃) + ... + (b_k-1 - a_k) + b_k
      b_k - a₁ b - a

Therefore, for any finite collection of open intervals I_n we have:

(**)      l(I_n) b - a

Now take an arbitrary collection of intervals I_n that cover [a, b]. By the Heine-Borel theorem we can extract a finite subcover, i.e. a finite number of intervals that still cover [a, b]. We already know that for the finite subcover inequality (**) holds, and the sum for all intervals in the cover is even greater than the left side of (**). Therefore (**) holds for any collection of open sets covering [a, b], and thus

m^*([a, b]) b - a

That together with (*) show that m^*([a, b]) = b - a, as required.

Interactive Real Analysis, ver. 2.0.1(b)
(c) 1994-2017, Bert G. Wachsmuth
Page last modified: Mar 3, 2017