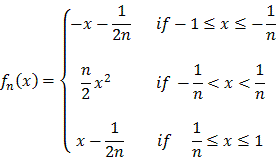Interactive Real Analysis - part of MathCS.org

Next | Previous | Glossary | Map | Discussion

Example 8.2.6 (b): Uniform Convergence does not imply Differentiability

Find a sequence of differentiable functions that converges uniformly to a continuous limit function but the limit function is not differentiable

Before we found a sequence of differentiable functions that converged pointwise to the continuous, non-differentiable function f(x) = |x|. Recall:

That same sequence also converges uniformly, which we will see by looking at ` || fn - f||D. We will find the sup in three steps:

If 1 x -1/n:
| fn(x) - f(x)| = |-x - 1/2n + x| = 1/2n

If -1/n < x < 1/n:
|fn(x) - f(x)| |n/2 x2| + |x| n/2 1/n2 + 1/n = 3/2n

If 1/n x 1:
| fn(x) - f(x)| = |x - 1/2n - x| = 1/2n

Thus, || fn - f||D < 3/2n which implies that fn converges uniformly to f. Note that all fn are continuous so that the limit function must also be continuous (which it is). But clearly f(x) = |x| is not differentiable at x=0.

Next | Previous | Glossary | Map | Discussion