C(n) Function
Why these ads ...
The function
g is
n-times differentiable, the
n-th derivative is continuous, and
g is not
(n+1)-times differentiable.
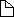
Back
|
On the right are the graphs of this root function for n = 0, 1
and 2. Can you decide which graph goes with what n ?
Click on Options and choose different powers of
x to see how the function gets smoother and smoother at
0, which is necessary if it wants to be more and more times
differentiable.
|
|
Proof:
This proof is left as an exercise. As a hint, start with
n = 1.
The example, if correct, says that for
n = 1 the function
g is
once differentiable, the derivative is continuous, but not differentiable
again. Why ? Then try
n = 2. Then, maybe, try an induction argument
to prove the general case.
Interactive Real Analysis
- part of