Interactive Real Analysis
- part of Examples 4.2.18(a):
The sum
converges conditionally.
Back
converges conditionally.
Back
We already know that the series does not converge absolutely (why ?). As for convergence, let us verify the conditions for Abel's test:
First, let
{ a n } = { (-1) n }and
{ b n } = { 1 / n }Then the sequence of partial sums of a n's is clearly bounded (by what number ?), and the sequence { b n } is decreasing and convergent to zero. Hence, Abel's test applies, showing that the series converges.
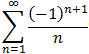Therefore, the series converges conditionally.