Corollary 8.3.11: Power Series is infinitely often Differentiable
If a power series
f(x) = 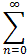 an (x-c)n
has radius of convergence r, then f is
infinitely often differentiable for |x-c| < r. In
other words,
f
an (x-c)n
has radius of convergence r, then f is
infinitely often differentiable for |x-c| < r. In
other words,
f  C
C (c-r, c+r)
.
(c-r, c+r)
.
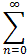 an (x-c)n
has radius of convergence r, then f is
infinitely often differentiable for |x-c| < r. In
other words,
f
an (x-c)n
has radius of convergence r, then f is
infinitely often differentiable for |x-c| < r. In
other words,
f  C
C (c-r, c+r)
.
(c-r, c+r)
.
By our previous theorem a power series with radius of convergence
r = lim sup |an|/|an+1|
can be differentiated term by term, so that
f'(x) =n an (x-c)n-1
But the center of this power series is again c and the radius of convergence is also
lim sup |n an|/|(n+1) an+1| = lim sup n/(n+1) |an|/|an+1| = r
Thus, the derivative itself is a power series with center c and radius of convergence r. Thus, by the same theorem, it can be differentiate again ... and again ... and again ...

 Interactive Real Analysis
- part of
Interactive Real Analysis
- part of