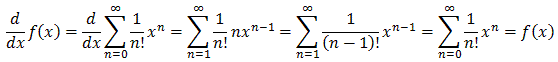Example 8.3.12 (a): Differentiating and Integrating Power Series
Find the derivative of the series
f(x) = 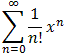 .
What is the radius of convergence? Make sure to simplify your answer, which
should be surprising. Can you use your result to figure out what function
this power series represents?
.
What is the radius of convergence? Make sure to simplify your answer, which
should be surprising. Can you use your result to figure out what function
this power series represents?
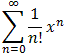 .
What is the radius of convergence? Make sure to simplify your answer, which
should be surprising. Can you use your result to figure out what function
this power series represents?
.
What is the radius of convergence? Make sure to simplify your answer, which
should be surprising. Can you use your result to figure out what function
this power series represents?
The radius of convergence turns out
r =  (confirm!). Thus,
the series can be differentiated term by term:
(confirm!). Thus,
the series can be differentiated term by term:
So the function defined by this power series is its own derivative! We know, of course, that there is only one function with that property, namely the expontential function (can you prove that?).
Thus, we have:
ex =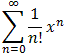
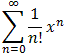
f(x) = ex
 Interactive Real Analysis
- part of
Interactive Real Analysis
- part of