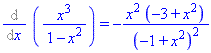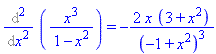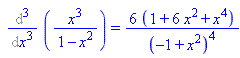Interactive Real Analysis - part of MathCS.org

Next | Previous | Glossary | Map | Discussion

Example 8.4.2 (c): Derivatives of Power Series

Find the 10th and 11th derivative at zero for the function f(x) = x3/1-x2. As a hint, try to get the geometric series function involved somehow.

The obvious first try is to take 10 derivatives, then substitute x=0. Let's take a few derivatives:

This is getting out of hand - the derivatives are getting more and more complicated. Being notoriously lazy, the good mathematician looks for a short-cut. The hint mentions the geometric series ... recall

1/1-x = 1 + x + x2 + x3 + ...

Substituting x2 and multiplying by x3 gives us:

x3/1-x2 = x3(1 + x2 + x4 + x6 + ... ) =
     = x3 + x5 + x7 + x9 ...

Comparing this with the coefficients of a power series we get:

a0 = a1 = a2 = 0
a3 = a5 = a7 = ... = 1
a4 = a6 = a8 = ... = 0

Since an = , or equivalently f (n)(c) = n! an, we have our answer:

  • f (10)(0) = 10! a10 = 0
  • f (11)(0) = 11! a11 = 11! = 39,916,800
Next | Previous | Glossary | Map | Discussion