Examples 4.2.16(e):
According to the ratio test the series
an/n!
converges absolutely, as you can readily check. But then, by the divergence theorem, the sequence
|a|n/n!
must converge to zero.
This is a pretty round-about proof with heavy artillery. A simple induction proof might be successful, too.
 Interactive Real Analysis
- part of
Interactive Real Analysis
- part of 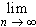 an/n! = 0
an/n! = 0