Examples 4.2.8:

Use the Cauchy Condensation criteria to answer the following questions:

In the sum 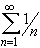, list the terms a ₄, a _k, and a _{2 ^k}. Then show that this series (called the harmonic series) diverges.
For which p does the series 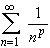converge or diverge ?

Back

The sequence { ¹/_n } corresponds to the harmonic series. Therefore:

a ₄ = 1/4
a _k = 1/k
a _{2 ^k} = 1 / 2 ^k

As for convergence or divergence of this series, we already know, by 'elementary' means, that this series diverges. Here is an alternative proof of this, using the Cauchy Condensation test as follows:

and the last series diverges by the Divergence test. Hence, the original series also diverges.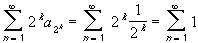

Next, we investigate the series 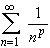for various p:

If p < 0 then the sequence 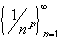converges to infinity. Hence, the series diverges by the Divergence Test.
If p > 0 then consider the series
=
The right hand series is now a Geometric Series, so that:
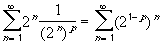
- if 0 < p 1 then 2 ^1-p 1, hence the right-hand series diverges
- if 1 < p then 2 ^1-p < 1, hence the right-hand series converges

But this is exactly what we need, in conjunction with Cauchy's Condensation test, to finish the proof of the statement.

Interactive Real Analysis, ver. 1.9.5
(c) 1994-2007, Bert G. Wachsmuth
Page last modified: Mar 26, 2007