MathCS.org - Real Analysis: Examples 4.2.4(a):

Examples 4.2.4(a):

The series

converges.

Back

Because | sin(x) | 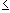1 for all real number x, we have that

1 / 2 ⁿ sin(n) | | 1 / 2 ⁿ |

But

is a special case of the geometric series, which converges. Hence, by the comparison test, the original series

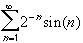converges absolutely.

Note that, formally, we could say: Since

| 1 / 2 ⁿ sin(n) | | 1 / 2 ⁿ |

for all n. We also have

| | <

"Therefore", the series converges absolutely.

Next | Previous | Glossary | Map | Discussion